

We then write out a logical order of Statements and their Reasons to prove that ∠1 and ∠2 are complementary. Like in two-column proofs, the first sentence in a paragraph proof is restating what we already know. Thus by the definition of complementary angles, ∠1 and ∠2 are complementary. Since ∠1 ≅ ∠3, ∠1 = ∠3 by the definition of congruent angles. By the Angle Addition Postulate, ∠WXY=∠2 + ∠3. Since ∠WXY is a right angle, ∠WXY=90° by the definition of a right angle. Using what we know about Paragraph Proofs, let’s evaluate the problem below… That’s why this type of proof is more commonly used at the collegiate level of geometry. Because paragraph proofs are so wordy, it can be hard for geometry beginners to understand them. Each Statement and its corresponding definition, theorem, property, or postulate needs to build off the previous one. Paragraph proofs need to be written so that each step supports the next. But instead of listing steps in columns, all the information is written out as a paragraph. Just like two-column proofs, they consist of multiple steps and each step contains a Statement and a Justification. Paragraph proofs are detailed paragraphs that explain the entire process of proving each Statement.
Because two column proofs are so easy to understand, high school teachers often use this proof as a way of introducing students to new geometric concepts. By combining both visual and written elements of geometry, you’re able to see and conceptualize why each written step is true. Two-column proofs present each step in an organized and concise manner. Review definitions, postulates, properties, and theorems to find Justifications that relate to each of your Statements. We then need to find a Justification that proves each of these problems true. To logically arrive at the conclusion that angle A is congruent to angle B, each step in the Statement column needs to be broken down into an algebra problem. Justifications can be geometric definitions, postulates (commonly accepted concepts based on mathematical reasoning), properties (a characteristic that applies to a given set of numbers), or theorems (rules that are demonstrated using formulas).
GEOMETRY THEOREMS LIST SERIES
So in order to prove it, we fill the Reasons column with a series of Justifications.

When you look at the problem it’s intuitive to assume that angle A is congruent to angle B, but we don’t know why they’re congruent. Next, we write down what steps we can take using Definitions and Properties to prove that ∠A ≅ ∠B. The first step is filling in the information we’ve already been given. ∠A and ∠C are complementary, ∠B and ∠C are complementary.Now we must prove that angles A and B are equal. It’s also given that B and C are complementary.

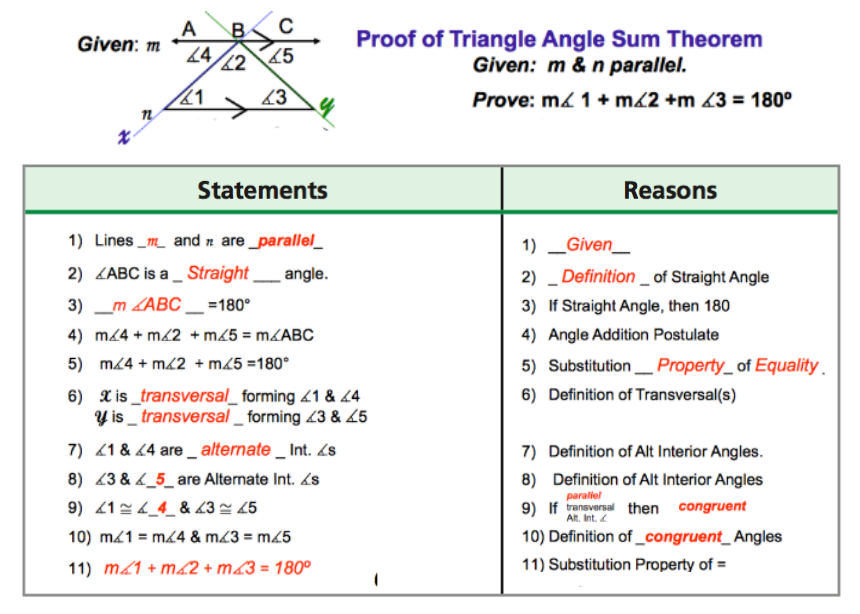
It’s given that C and A are complementary, meaning that when you add them together they equal 90°. The second has a list of Reasons that correlate to each Statement. Two-column proofs are a type of geometric proof made up of two columns. So here is a breakdown of three of the most useful geometric proofs, how and when to use them, and why knowing them will make geometry so much easier! Two-Column Proofs Each method provides a different way to list the steps and show why each Statement is true.
GEOMETRY THEOREMS LIST HOW TO
Today we will demonstrate how to write a proof using columns, boxes, and paragraphs. Reasons are pieces of evidence that support a Statement. Statements are claims about a geometric problem that cannot be proven true until backed by a mathematical Reason. Geometric proofs are a list of Statements and Reasons used to prove that a given mathematical concept or idea is true. In order to do this, you must utilize geometric proofs. Unlike other types of math, in geometry you’re often given the answer to a problem and asked to demonstrate how it’s true. Much of geometry is about working backwards in order to solve problems.


 0 kommentar(er)
0 kommentar(er)
